Using Excel

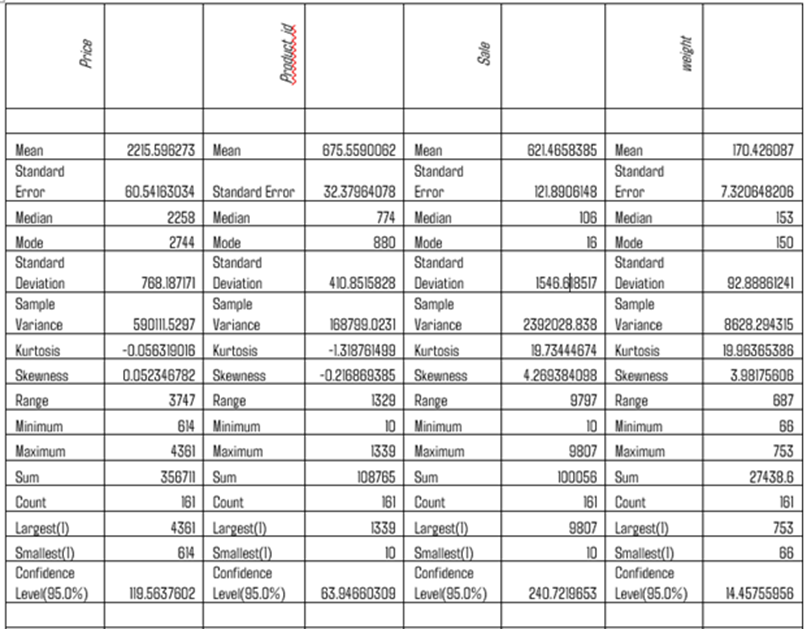
Descriptive Statistics

Go to excel >> data menu >> data analysis

Calculation of all beta coefficients using matrix in excel

Same problem. We have 161 observations and 14 variables. Price is Dependent and all others (13) are independent variables. Now add new column in front of productid column and fill with 1 as shown below. That all the first column must be filled with 1 (cover 161 rows)

	Product_id	Sale	weight	resoloution	ppi	cpu core	cpu freq	internal mem	ram	RearCam	Front_Cam	battery	thickness	Price
1	203	10	135	5.2	424	8	1.35	16	3	13	8	2610	7.4	2357
1	880	10	125	4	233	2	1.3	4	1	3.15	0	1700	9.9	1749
1	40	10	110	4.7	312	4	1.2	8	1.5	13	5	2000	7.6	1916
1	99	11	118.5	4	233	2	1.3	4	0.512	3.15	0	1400	11	1315
1	880	11	125	4	233	2	1.3	4	1	3.15	0	1700	9.9	1749
1	947	12	150	5.5	401	4	2.3	16	2	16	8	2500	9.5	2137
1	774	13	134.1	4	233	2	1.2	8	1	2	0	1560	11.7	1238

Polynomial models can also be considered as multiple linear regression models

Multiple linear regression can be written in the matrix form

model betas error

Our aim is to minimize sum of squares

Minimize sum of squares

First, we have to which are variables are independent and which one is dependent variables. In our case Price is dependent variable y and X are other independent variables. As explained earlier add a new column in front of all independent variable. X matrix must contain 1 in the first column

In excel we have to find our the following

X – Cell range from Product_Id to thickness AND assign a name as X
Xt Formula used is “=TRANSPOSE(X)” AND assign a name as Xt
XtX Multiplication of X(transpose)*X Formula used : “=MMULT(Xt,X)”
XtXinv Inversion of XtX Formula used: “=MINVERSE(XtX)”
Xty Multiplication of X(transpose)*y Formula used ; “=MMULT(Xt,y)
Beta = “MMULT(XtXinv,Xty)

X

Select the data from A2 to N162 and name the table as X

Y

Select Price column from O2 to O162 and name the table as y

Xt(transposeX) –>(=Transpose(X))

Select X variables including the first column. For output Fourteen rows and 161 columns are selected before passing the command =TRANSPOSE(X). Values goes up to from Q to FU. We have not shown the values from AK to FU columns.

Now Select the columns from Q3 to FU16 and name the table as Xt

Multiplication of Xt and X

XtX -> (MMULT(Xt,X)

XtX
161	108765	100056	27438.6	838.8	53944	782	241.956	3944.776	355.004	1670.9	725	457580	1436.4
108765	100485019	89813290	18755415	564890.36	38279394	526900	167099.8	3159490.11	264821.3	1199014	510051.6	311974910	976110.4
100056	89813290	444906000	17422562	527695.23	41394818	528030	165080.8	5939330.72	369264.3	1485328	920361.1	325077695	866635.1
27438.6	18755415	17422562	6056780	162935.41	9083890.8	141128.4	43221.05	714610.268	64073.63	282059.5	123173.6	94923082	238763
838.8	564890.36	527695.23	162935.4	4734.8892	286950.12	4353	1333.818	21962.3488	1976.811	9078.48	3989.86	2662520.6	7200.846
53944	38279394	41394818	9083891	286950.12	20982772	287742	90296.24	1706083.64	144947.7	663059.5	288941.2	162235820	457776.8
782	526900	528030	141128.4	4353	287742	4754	1290.496	22272	2028.44	9593.6	4517.6	2468280	6378.4
241.956	167099.76	165080.84	43221.05	1333.8175	90296.236	1290.496	421.1776	7148.48	631.3869	2881.905	1238.86	749733.8	2029.38
3944.776	3159490.1	5939330.7	714610.3	21962.349	1706083.6	22272	7148.48	229408.164	15192.75	53814.78	28884.8	14119043.6	31481.56